Jonathan A. Sherratt, Department of Mathematics, Heriot-Watt University

Modelling the Movement of Interacting Cell Populations

Mathematical modelling of cell movement has traditionally focussed on a single population of cells. However, many biological systems actually involve two or more cell populations. In collaboration with Kevin Painter, I have developed a simple partial differential equation model for this situation.

A motivating example

 Imagine a confluent sheet of cells, for example in a petri dish. If a strip of cells is removed from the sheet, the cells on either side of the gap will migrate and divide in order to fill the gap. 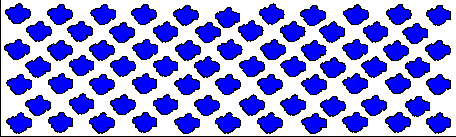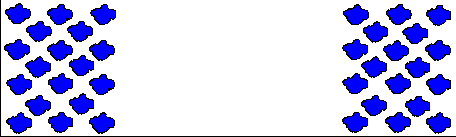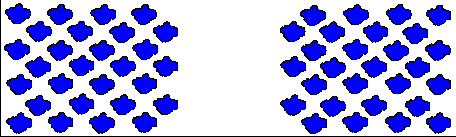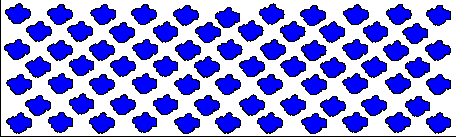(This is a very simple example of wound healing). The figure illustrates this process of gap creation following by healing. As the cell populations on either side of the gap come together, they will not intersperse significantly, because the cells stop moving when they meet ("contact inhibition"). This is illustrated in the figure by pointing to it with the mouse. The cell populations on either side of the gap appear in different colours. This could be acheived experimentally by appropriate cell staining. Mathematically it is straightforward to formulate a partial differential equation for the overall cell population. (The Fisher equation is a good model, for example). But there is no standard model in which the red and blue cell populations can be represented by different variables. The objective of our work was to develop such a model.

Equations based on space jump probabilities

 Illustration of transition probabilities 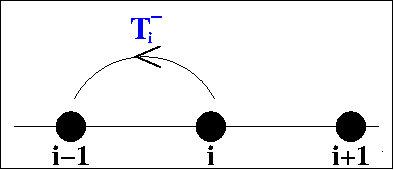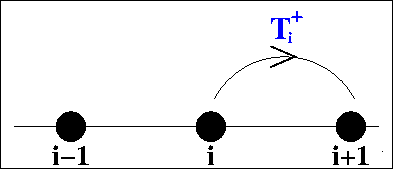Kevin Painter and I dervied our model using "jump probabilities". Assume that the cells move on a one-dimensional lattice, with T-i / T+i being the probability of moving from site i to site i-1/i+1. Then it is straightforward to derive ordinary differential equations for the cell density at each site and to then derive a corresponding partial differential equation by taking an appropriate limit. The equations vary according to the dependence of the transition probabilites on the cell densities. The assumption that T+i and T-i are independent of cell density gives separate linear diffusion terms for the two populations, which would imply mixing of the red and blue populations in the schematic figure above. But it is more realistic to assume that the probability of the cell moving to the left/right is a decreasing function of the cell density at the potential destination. With suitable functional forms, this gives the model equation and solutions show below.
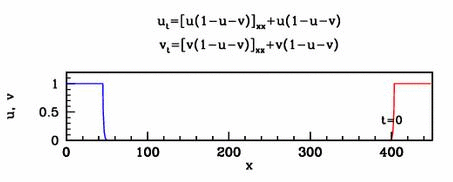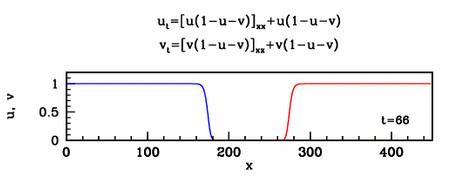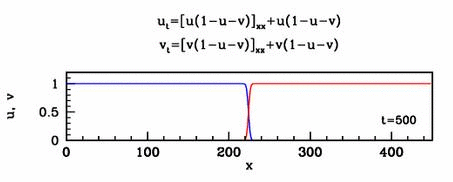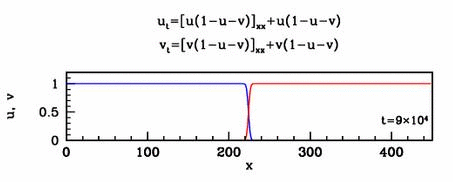
Illustration of transition probabilities

Applications

 In collaboration with Mark Chaplain, I have applied a simple form of the new model to early tumour growth. Many other potential applications in developmental biology and physiology remain to be explored.

Travelling waves

 The nonlinear diffusion in the new model leads to unusual travelling wave behaviour. For one special case of the new model, I have studied this in detail. I showed that there are travelling wave solutions for all speeds above a critical minimum value, a phenomenon familiar from simple reaction-diffusion equations such as the Fisher equation. However for this new model, I showed that the minimum speed arises through a new and quite different mathematical mechanism.

The work described on this page is discussed in the following papers:

Back to Jonathan Sherratt's Research Interests
Back to Jonathan Sherratt's Home Page